
Home | Search | About | Fidelio | Economy | Strategy | Justice | Conferences | Join
Highlights | Calendar | Music | Books | Concerts | Links | Education | Health
What's New | LaRouche | Spanish Pages | Poetry | Maps
Dialogue of Cultures
Bernhard Riemann’s
‘Dirichlet’s Principle’
This article is reprinted from the Winter 2000FIDELIO Magazine.
For related articles, scroll down or click here.
 Fidelio, Vol. XIII,
Fidelio, Vol. XIII,No, 4. Winter 2004
Bernhard Riemann's 'Dirichlet's Priniciple'
In his revolutionary essay of 1857, Theory of Abelian Functions, Bernhard Riemann brought to light the deeper epistemological significance of the complex domain, through a new and bold application of a principle of physical action which he called “Dirichlet's Principle.” Riemann's approach, combined with what he enunciated in his habilitation dissertation of 1854, not only ushered in a revolution in scientific thinking: it ignited a counter-reaction as fierce as the one launched, for the same reasons, against Nicolaus Cusa, Kepler, Fermat, and Leibniz by the Venetian-British-controlled empiricist school of Galileo, Newton, Euler, and Lagrange, a counter-reaction that continues to rage to this day, with implications that reach far beyond the specific setting of Riemann's 1857 paper. Despite the volumes that have been written on this subject, from Riemann's time to ours, an honest examination of the history of the matter reveals that, just as Gauss demonstrated the fraud of Euler, Lagrange, and D'Alembert in his 1799 proof of the Fundamental Theorem of algebra, Riemann was right, and his critics, like today's Straussian controllers of Bush and Cheney, were malevolent frauds.
We cannot know for sure whether, when Riemann chose to call this method an application of “Dirichlet's Principle,” he expected to provoke the reaction he received, or if he was merely stating what would have been obvious to anyone within the extended network of Abraham Kästner's students. Nevertheless, it is fortunate for us that he used that name, as it enables us to fairly accurately reconstruct, not only the scientific origins of Riemann's thought, but the historical-political process from which it arose.
Enter Lejeune Dirichlet
Johann Peter Gustav Lejeune Dirichlet was a pivotal figure in early Nineteenth-century science. Born in 1805 to a family of Belgian origin living near Aachen, his early education took place in Bonn. At the age of 16, with a copy of Gauss's Disquisitiones Arithmeticae under his arm, he went to Paris to audit lectures at the College de France and the Faculté des Sciences. After a year, Dirichlet became employed as a tutor by General Maximilien Sebastien Foy, a republican member of the Chamber of Deputies, who introduced him to Alexander von Humboldt. After Foy's death in 1825, von Humboldt recruited Dirichlet to return to Germany, arranged for him to get a degree (even though Dirichlet refused to speak Latin), and eventually succeeded in obtaining for him a professorship at the University of Berlin. There, in addition to meeting, and marrying, Moses Mendelssohn's granddaughter Rebecca (a sister of the composer Felix Mendelssohn), Dirichlet developed a fruitful collaboration with Karl Jacobi and Jakob Steiner, including touring Italy with both in 1843 under Alexander von Humboldt's sponsorship.
In 1847, Riemann arrived in Berlin to study with Dirichlet, Jacobi, and Steiner, having spent the previous two years studying with Gauss. In 1849 he returned to Göttingen to complete his studies, and in 1851, under Gauss's direction, published his doctoral dissertation, “The Foundations for a General Theory of Functions of a Complex Variable Magnitude,” in which he for the first time applied his principle, without mention of Dirichlet. When Gauss died in 1855, Dirichlet was appointed his successor, bringing himself back into contact with Riemann, who had received permission to teach just seven months earlier, after delivering his habilitation lecture, “On the Hypotheses Which Lie at the Foundations of Geometry.” In 1857, Riemann published the Theory of Abelian Functions, in which, for the first time, he identified as “Dirichlet's Principle,” the principle on which his new theories were based. Dirichlet died two years later, and Riemann, now 33 years old, was appointed to Dirichlet's chair, a position he held until his own premature death only seven years later.
The Potential
What Riemann called “Dirichlet's Principle,” arose out of Gauss's application of the complex domain to his investigations in geodesy and terrestrial magnetism, the former organized in collaboration with Heinrich Schumacher beginning in 1818, and the latter initiated by Alexander von Humboldt in 1832. Both projects had enormous practical benefits. Each produced detailed maps of their respective physical effects, which were vital for infrastructure development, and Humboldt's project organized, for the first time, an international collaborative network of scientists that would have an impact on the development of the physical economy from the Americas to Eurasia for generations. But, Gauss recognized that both projects posed deeper epistemological questions for science. Writing in his General Theory of Earthmagnetism in 1839, Gauss said that a complete and accurate map of the observations was not, in itself, a proper goal for science, since “one has only the cornerstone, not the building, as long as one has not subjugated the appearances to an underlying principle.” Citing the case of astronomy as an example, Gauss said that mapping the observations of the apparent motions of the heavenly bodies onto the celestial sphere, was just a beginning: Only once the underlying principle of gravitation was discovered, could the actual orbits of the planets be determined.
Gauss recognized that the first step in both geodesy and geomagnetism was the measurement of changes in the effects both phenomena had on the measuring instruments. In the case of geodesy, this meant changes in the direction of a plumb bob, or plane level, as those changes were mapped onto the celestial sphere. The case of geomagnetism is more complicated. Here, changes in the direction of a compass needle were being measured, with respect to three directions and time. The general question was: What is the characteristic nature of the principle of gravitation, or geomagnetism, that would produce these apparent effects? The specific task was: How, from these infinitesimally small, measured changes in the apparent effects, can that general characteristic be determined?
It is the second question which brings us more directly into contact with what Riemann called “Dirichlet's Principle.” However, the task of understanding “Dirichlet's Principle” will be made much easier, if we first look at the elementary, but congruent case of the catenary.
The relevant focus for this discussion is the devastating rebuke which Leibniz and Bernoulli delivered to Galileo and Newton over the case of the catenary. Galileo had insisted that all that needed, or could, be known about the catenary, was a description of its visible shape. On the other hand, Leibniz and Bernoulli insisted that the shape of the catenary was merely the visible effect of an underlying physical principle, and that the correct shape could not be determined until the underlying principle was known. As has been developed in previous Pedagogical Exercises,1 Leibniz and Bernoulli determined the characteristic nature of that principle, by first determining the changing physical effect of that principle in the infinitesimally small, and then, by inversion, the overall characteristic of the principle. The result was Leibniz's discovery that the shape of the hanging chain reflected the least-action effect of the principle of universal gravitation, and that this effect could be expressed geometrically as the arithmetic mean between two contrariwise exponential functions.2
It is of extreme importance to emphasize that we are speaking here of the physical hanging chain, and not a formal mathematical expression. In a formal mathematical expression, the exponential curves have no boundary. But the physical hanging chain does—the positions of the hanging points; consequently, the specific shape of the chain is determined by the positions of the hanging points relative to the weight and length of the chain. If the positions of the hanging points change, the position of every link in the chain also changes, albeit always in accordance with the relationship cited above. In other words, as the boundary conditions of the physical chain change, so does the specific path of the chain, but that path's general form, required by the principle of least-action, is always a catenary. It will never become a parabola or any other curve [ Figure 1].
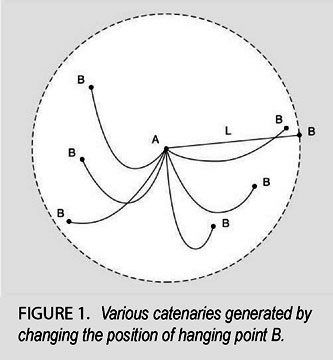 |
||
This example illustrates an aspect of the method that Leibniz originally called “analysis situs”—or what Gauss and Carnot later called “geometry of position”—that is relevant to an understanding of Riemann's “Dirichlet's Principle.” The positions of the individual links in the chain are a function of the relationship of the boundary conditions (positions of the hanging points, relative to the length of the chain) to the characteristic curvature of the principle of gravitation, and not by pair-wise relationships among the links themselves. In other words, the position of any individual link is not determined by a distance to the right or left, and a distance up or down, from its neighbors, as the Cartesians and Newtonians would insist. Rather, the position of each link is a function of the characteristic of change of the physical action as a whole. Any change in the boundary conditions, changes the position of every link, as a whole in conformity with the least-action principle of the catenary. Thus, the effect in the visible domain of the unseen physical principle, is expressed by the characteristic of change demanded by the principle of least-action. This is what determines the specific positions of the links. In other words, position is a function of change.
Gauss recognized that the principles underlying geodesy and geomagnetism could be understood by an extension of Leibniz's method. He rejected the popularly accepted, but provably false method of Newton, which attempted to explain these phenomena as resulting from the pair-wise interaction of material bodies, according to the algebraic formula of the inverse square.3 Instead, Gauss insisted that these phenomena, as in the case of the catenary, must be understood as a unified process, in which the local variations in the position of the plumb bob or compass needle were a function of the characteristic of the principle governing the phenomenon as a whole. That whole, Gauss called “the potential,” which is the Latin equivalent of the Greek “dynamis,” or Leibniz's “kraft” (or Latin “vis viva”). Gauss invented the idea of a “potential function,” to express the least-action effect of the physical principle over an area or volume, in a similar, but extended manner to that used by Leibniz to express the effect of gravity in producing the curvature of the hanging chain. To accomplish this, Gauss extended Leibniz's idea of a function, into the complex domain.
This transformed Leibniz's functions—which characterized a single minimal pathway—into Gauss's “potential function,” which characterized a whole class of minimal pathways: in effect, a function of functions. In other words, if Leibniz's catenary is understood to be a minimal pathway determined by one set of two functions, Gauss's potential function takes the next step, to a function that unifies two (or more) sets of functions. Riemann would later show that these sets of minimal pathways implicitly define minimal surfaces, as, for example, the catenoid formed by a soap film suspended between two circular rings [Figure 2.]
 |
||
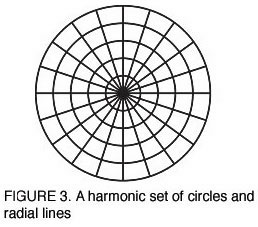
These sets of functions are not arbitrary. They are related by a special type of relationship, called by the descriptive names “spherical” or “harmonic” functions. A spherical or harmonic function is a set of orthogonal functions, all of whose curvatures are changing at the same rate.
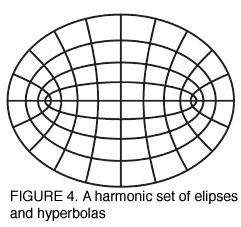
This can most easily be illustrated pedagogically with some geometric examples. A set of concentric circles and radial lines composes an harmonic function, because both the circles and the radial lines intersect orthogonally, and both have constant curvature [Figure 3]. A more illustrative example is a set of orthogonal ellipses and hyperbolas [Figure 4].
 |
 |
|
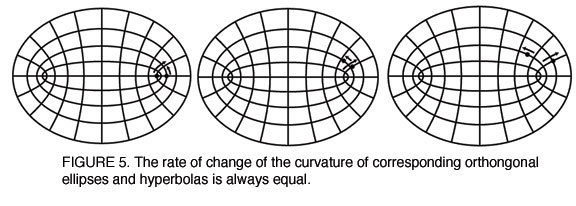
To get an intuitive grasp of their harmonic relationship, think through the following. Each ellipse is associated with a confocal orthogonal hyperbola. Beginning at the point where both curves meet the axis, create in your mind a connected action that moves simultaneously on both curves [Figure 5].
 |
|
Note that, as the curvature on the hyperbola becomes less curved, so does the curvature on the corresponding ellipse, and at the same rate.
Thus, harmonic functions relate two sets of different curves, such that the rate of change of their respective curvatures is always equal. (We could calculate this relationship precisely using Leibniz's calculus, but an intuitive understanding is sufficient for present purposes.)
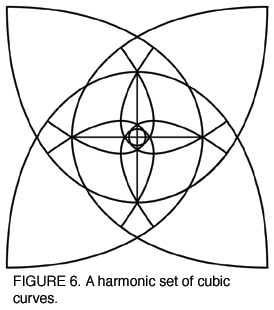
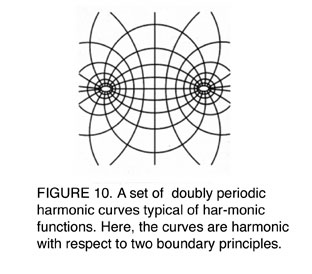
Furthermore, a set of harmonic functions need not be of familiar curves, such as circles, lines, ellipses, or hyperbolas. In fact, very complicated sets of functions can be harmonic [Figure 6].
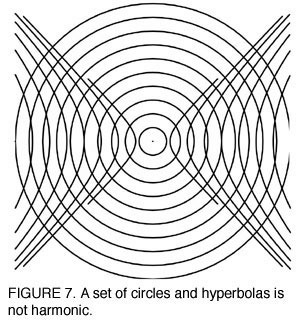
By contrast, a set of circles and hyperbolas is not harmonic, because the curvature of the circle is constant, while the curvature of the hyperbola is changing. Consequently, the two sets of these curves are not orthogonal [Figure 7.]
 |
 |
|
Gauss recognized that Leibniz's principle of least-action with respect to the surfaces and volumes encountered in phenomena like terrestrial gravitation and magnetism, could be expressed by harmonic functions. One set of curves of the harmonic function expressed the pathways of minimal change in the potential for action, while the other, orthogonal curves expressed the pathways of maximum change in the potential for action. For example, if the Earth were perfectly spherical, its minimum and maximum of potential action could be expressed by a series of concentric spherical shells and orthogonal planes. A cross-section of such a configuration would be harmonically related circles and radial lines. If the Earth were perfectly ellipsoidal, its potential would be expressed by a set of triply orthogonal ellipsoids and hyperboloids whose cross section would be the harmonically related set of ellipses and hyperbolas illustrated in Figure 4.
But, as Gauss emphasized, the shape of the Earth is much more complicated than a sphere or an ellipsoid, with respect to both gravity and magnetism, and the pathways of minimal and maximal potential for action are not such simple and well-known curves as circles, lines, ellipses, or hyperbolas. Thus, a more complex harmonic function must be found, to express these principles. Such a function could not be determined a priori, but only from the measured changes in the effect of the Earth's gravity or magnetism.
The question for Gauss was: How to determine the true physical shape of the Earth, or the characteristic of the Earth's magnetism, from the measured, infinitesimally small changes in its potential obtained by his geodetic and magnetic measurements?
This begins to get us closer to a first approximation of what Riemann called “Dirichlet's Principle.”
To make a precise determination of the Earth's surface, or magnetic effect, as Gauss did, is quite complicated, but the principle on which his method was based is within the scope of this Pedagogy. If one recognizes, as Gauss did, that changes in the direction of the plumb bob are measuring changes in direction of the potential function, then the physical shape of the Earth has the same relationship to this potential, as the hanging points have to the catenary. In other words, the surface of the Earth must be understood as merely the boundary of the potential, or, as Gauss put it, “the physical surface of the Earth is, in a geometric sense, the surface that is everywhere perpendicular to the pull of gravity.”
A reference to the ancient Pythagorean problem of doubling the line, square, or cube, can shed some light on this idea. The line is bounded by points, the square by lines, and the cube by squares. The size and position of these boundaries is determined by the length, area, or volume they enclose. For example, it is the square that determines the size and position of its sides, even though it is the latter that you see, and the former that you don't. The sides of the square are lines, but they are produced by a different power (potential), than the lines produced from other lines. Similarly, the size and position of the squares that form the boundaries of a cube are produced by a different power (potential), than the squares formed by the diagonal of another square. Thus, even though the power can not be seen, it can be measured by its unique, characteristic effect on the boundaries of its action.
Now, apply this same method of thought to the physical principles discussed above. The catenary is a curve whose boundaries are points. A catenoid is a surface whose boundaries are curves. The surface of the Earth is the boundary of a gravitational volume. The magnetic effect of the Earth is still more complicated, and will be taken up in more detail in a future Pedagogical.
This connected relationship between the boundary conditions of a physical process, and the expression of the principle of least-action with respect to that physical process, is the relationship to which Riemann is referring when he speaks of “Dirichlet's Principle.”
From Gauss, to Dirichlet, to Riemann
After succeeding Gauss in 1855, Dirichlet began lecturing on Gauss's potential theory at Göttingen, while Riemann was preparing his Theory of Abelian Functions. What Gauss, Dirichlet, and Riemann all recognized was, that complex functions, as the extension of Leibniz's concept of the catenary and natural logarithms, were uniquely suited to express the least-action pathways of potential functions.
Gauss had already demonstrated this in his 1799 proof of the Fundamental Theorem of algebra, where he showed that a complex algebraic expression produces two surfaces whose curvatures are harmonically related. What Riemann attributed to Dirichlet, was the principle that given a certain boundary condition, the function that minimizes the action within it is a complex harmonic function.
Warm up to this idea on the familiar territory of the catenary. The boundary conditions here are the positions of the hanging points. The “interior” of this boundary is the curve itself. Within the curve there is a singular point—the lowest point. If the boundary conditions change, by changing the positions of the hanging points, so does the position of the lowest point. To state Dirichlet's principle in this simplified context, the catenary is the least-action pathway of a hanging chain with these specified boundary conditions and singularity. If the boundary conditions change, the shape of the curve changes correspondingly, in accordance with the preservation of the principle of least-action.
Riemann inverted Dirichlet's principle: Since the physical principle of least-action is primary, the position of the hanging points and the lowest point completely determine the shape of the chain!
Now, make this same investigation with respect to a catenoid formed by a soap film between two circular rings. This catenoid is a physical least-action, or minimal surface. Embedded in this surface is an orthogonal set of curves of minimal and maximal action. (Riemann later showed that these curves are harmonically related.) Experiment by changing the shape of these boundaries from circles, to ellipses, to irregular smooth shapes, to polygons. When you change the position or shape of the boundaries of this surface, the shape of the surface and the embedded curves change accordingly, but the least-action principle is preserved.
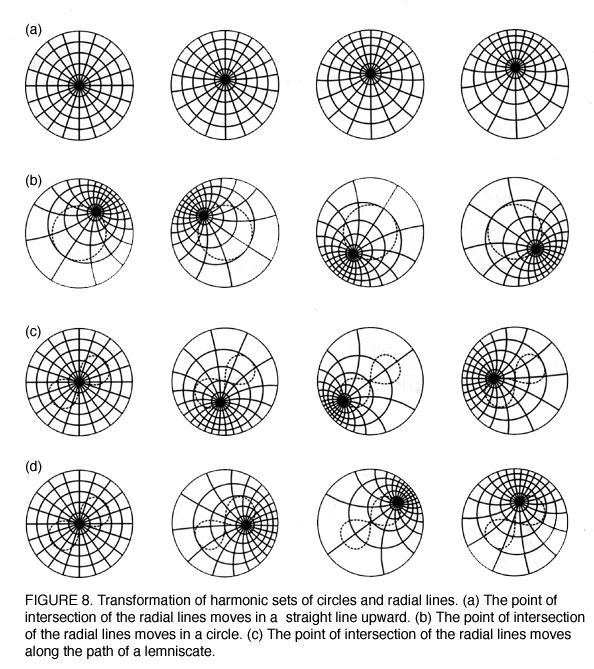
Now, generalize this idea with some other pedagogical examples, illustrated in the following figures derived from computer animations. In Figure 8 we see a set of harmonically related circles and
 |
|
radial lines that intersect at the center of the circles, being transformed while maintaining their harmonic relationship. If the position of that intersection point changes, the radial lines must be transformed into circular arcs, and their end-points move along the boundary in order to maintain their harmonic relationship. In the animation, this effect is shown as the point of intersection moves, first away from the center, and then in a circular path around the center. This motion causes all positions inside the boundary to change as a whole. What doesn't change is the harmonic, i.e., least-action, relationship.
This could also be thought of inversely: That the changes in position of the intersection of the radial lines at the boundary, cause their point of intersection to move in a circular arc, and their form to change from lines to circular arcs.
Or, infinitesimally small changes in the curvature of the pathways are determined by the conditions at the boundary with respect to the position of the singularity.
Compare this action with the change in the position of the lowest point of the catenary as the positions of the hanging points change, as illustrated in the animation Figure 1.
There, a change in the boundary points produced a change along a single curve. Here, a change in the boundary curve produces a change in a set of harmonically related curves within a surface.
Compare this with the problem Gauss confronted in, for example, determining the location of the Earth's magnetic poles from infinitesimally small changes in the Earth's magnetic effect. Gauss understood that those small changes were connected to the position of the singularities, i.e., magnetic poles, of the Earth's magnetic effect. However, the exact location, or even the number of those poles was still unknown in Gauss's time. On the basis of the measurements obtained by von Humboldt's network, Gauss determined where those poles must be located. The famous American Wilkes Expedition of 1837 was launched, in part, to confirm Gauss's findings, which it did.
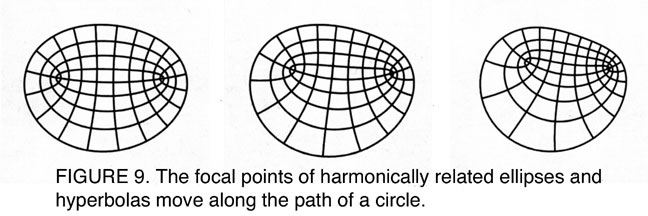
In Figure 9, this same effect is illustrated by moving the point of intersection of the radial lines along
 |
||
the path of a lemniscate. Notice again how this change in the position of the singularity, changes the condition at the boundary, so that all the resulting relationships remain harmonic.
 |
|
In short: A physical process of least action is a connected action. Changing any aspect of the process, changes everything else in the process correspondingly, so as to preserve the least-action characteristic of the process. It is the physical principle of least-action that is primary.
It was Riemann's genius to recognize, through this application of “Dirichlet's Principle,” that the principle of least-action of a physical process could be understood completely by the relationship between the boundary conditions and the singularities, and that this relationship could be expressed uniquely by Riemann's geometric concept of complex functions. Moreover, Riemann showed that the characteristic of least-action of a physical process could be changed, in a fundamental way, only by the addition of a new principle. That change in principle is expressed in a complex function, as a corresponding increase in the number of singularities. In his Theory of Abelian Functions, Riemann demonstrated this by applying “Dirichlet's Principle” to the higher, transcendental functions of Abel.
The deeper significance of this discovery can only be hinted at in this installment, and will be taken up in more depth later, but it can be illustrated by the animation illustrated in Figure 11, which expresses the principle of
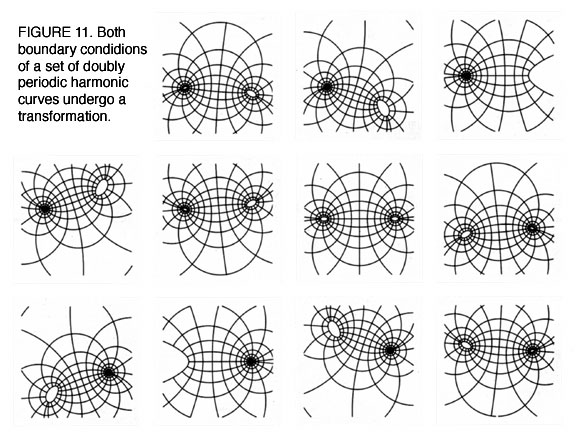 |
||
least-action with respect to an elliptical function. Riemann demonstrated that all elliptical functions, being functions formed by the interaction of two connected principles, are expressed in the complex domain as surfaces with two boundaries (these boundaries are marked in green). Each boundary changes differently, but
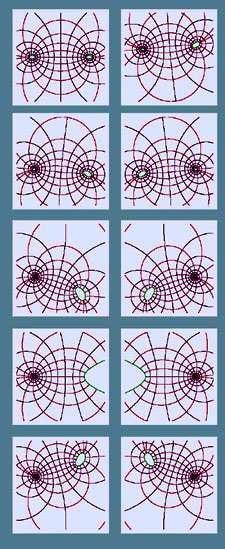 |
||
A comparison of this to the previous examples indicates what Riemann emphasized: That the only way to fundamentally change the characteristic of action of a physical process, is by the addition of the action of a new principle. This more advanced question will be investigated more thoroughly in future Pedagogicals.
A suggestive example from economics can help illustrate this principle. What is the relationship between all physical-economic relationships, and the economic boundary conditions of physical infrastructure and cultural development? What is the relationship between these boundary conditions, and the singularities represented by the introduction of new technologies? What is the effect on all economic relationships, of a change, positive or negative, in these physical-economic boundary conditions?
Four years after Riemann's death, Karl Weierstrass criticized Riemann's application of “Dirichlet's Principle” on formal mathematical grounds. Weierstrass contended that it was inappropriate to speak mathematically of least-action, unless a formal mathematical proof could be presented proving that a mathematical minimum, or maximum, existed. While it is possible to produce a formal mathematical example which has no minimum, all physical process are characterized by bounded least-action. For example, as Nicolaus of Cusa showed, there is no absolute maximum or absolute minimum polygon, because the polygon is bounded maximally by a circle (which is not a polygon) and minimally by a line (which is also not a polygon). Or, while a mathematical catenary can be extended into infinity, the physical one is always bounded by the hanging points. For Riemann, as for Gauss and Dirichlet, Weierstrass's demand for a formal mathematical proof of a minimum, was less than unnecessary: It was a sophistry. The universal physical principle of least-action was sufficient to supply the proof.
Weierstrass's critique was seized upon by the formalists, who were desperate to roll back the achievements of Kästner, Gauss, Dirichlet, Jacobi, Abel, Riemann, et al., and return science to the slavish days of Euler, Lagrange, and D'Alembert. Consequently, while the form of Riemann's discoveries has been widely discussed, the substance of his thinking has by and large been suppressed, until it found new life in the more advanced discoveries of Lyndon LaRouche.
—Bruce Director
|
Footnotes |
||
1. See, e.g., Bruce Director, “The Long Life of the Catenary: From Brunelleschi to LaRouche,” Fidelio, Spring 2003 (Vol. XII, No. 1).
2. See G.W. Leibniz, “Two Papers on the Catenary Curve and Logarithmic Curve (Acta Eruditorum, 1691),” trans. by Pierre Beaudry, Fidelio, Spring 2001 (Vol. X, No. 1).
3. See Bruce Director, Riemann for Anti-Dummies, Part 53: “Look to the Potential,” Dec. 21,2003 (unpublished).
|
Examples |
||
What is the Schiller Institute?
Pedagogy - Gauss's Fundamental Theorem of Algebra
Pedagogy - Hyberbolic Functions- A Fugue Across 25 Centuries!
Pedagogical Studies-- “Justice for the Catenary”
Schiller Institute 2004 Labor Day Conference Pedagogical Museum
Schiller Institute Pedagogy Articles
Fidelio Table of Contents from 1992-1996
Fidelio Table of Contents from 1997-2001
Fidelio Table of Contents from 2002-present
Beautiful Front Covers of Fidelio Magazine

Join the Schiller Institute,
and help make a new, golden Renaissance!
MOST BACK ISSUES ARE STILL AVAILABLE! One hundred pages in each issue, of groundbreaking original research on philosophy, history, music, classical culture, news, translations, and reviews. Individual copies, while they last, are $5.00 each plus shipping
Subscribe to Fidelio:
Only $20 for 4 issues, $40 for 8 issues.
Overseas subscriptions: $40 for 4 issues.
PO BOX 20244
Washington, DC 20041-0244
703-297-8368
Home | Search | About | Fidelio | Economy | Strategy | Justice | Conferences | Join
Highlights | Calendar | Music | Books | Concerts | Links | Education | Health
What's New | LaRouche | Spanish Pages | Poetry | Maps |
Dialogue of Cultures
© Copyright Schiller Institute, Inc. 2005 All Rights Reserved.