|
||||
Attack the Mathematicians To Solve the Economic Crisis |
||||
|
by Lyndon H. LaRouche, Jr.
Lecture at the Methodlogical University, Moscow June 8, 1995 |
||||
For related articles, scroll down or click here.
We Must Attack the Mathematicians
To Solve the Economic Crisis
by Lyndon H. LaRouche, Jr.
Nina Gromyko of the Methodological University introduced Mr. LaRouche as the founder of the science of physical economy, who is known in Russia through his textbook “So, You Wish to Learn All About Economics?,” which was published in its Russian edition in Moscow in 1993.
One should not exaggerate: I did not create the science of physical economy, I merely revived it. It started many years ago, back in the 1930’s, when I was in my adolescence (which almost is ancient history for some of you, perhaps). I took up the study of philosophy, of French, English, and German philosophy, from the Seventeenth and Eighteenth centuries, especially. And early on, I became a follower of Leibniz. Then I became an enemy of Kant, in defending Leibniz against Kant.
So in the later course of time, in the 1940’s, after the World War II, at the end of 1947 or the beginning of 1948, I met the work of Norbert Wiener, who has a certain reputation as the so-called “father of information theory,” which was becoming very popular. I should tell you that Norbert Wiener based his idea of information and human intelligence on gas theory, the statistical theory of gasses from Ludwig Boltzmann—and since then, you probably have heard, a great amount of gas has been issued on the subject of information theory!
I decided that this was the most disgusting thing I had ever seen, but I also recognized that what Wiener was saying, was merely a degenerate version of what Kant had already said. And, with the arrogance of a young man, I said, “I can defeat this. I could wipe the floor with this fool, Professor Wiener.” And I did, in a manner of speaking.
But out of this, in proving the nature of human scientific discovery, naturally I looked at the role of technology as typical of human ideas. And the use of language to communicate ideas about technology or scientific discovery, is the crucial proof, a very simple proof, in the sense of all the ideas of not only Wiener, but the ideas of an idiot-savant, who is a very skillful mathematician but an idiot-savant nonetheless, John Von Neumann. Von Neumann was a man who could fill blackboards in many buildings full of formulas in a single day, without ever presenting a single idea. He is the principal founder of what is called today “systems analysis,” which also eliminates any possibilities of ideas.
Once I had solved the problem, the question was, how should we attack the mathematicians? So I turned, first of all, to a study of the work of Georg Cantor, and, in the same year after studying Cantor, particularly his last major work, his Contribution on the Transfinite, I returned to read again the crucial discovery of Bernhard Riemann, and then I discovered why you cannot represent ideas mathematically, although you can present functions which explain with ideas what happens in mathematics.
I understand that some of you have been studying matters of formal logic. Well, let’s discuss it from the standpoint of formal logic.
To take a model of formal logic, instead of using “logic” in the sense it’s used today, or the Aristotelian syllogism, or metaphysics, let’s look at geometry. We don’t use an “equals” sign in logic any more. We will use “greater than” and “lesser than,” in several senses, and we will use the congruence sign rather than the “equals” sign. Because two things may appear to be equal, but they’re not congruent. We have many modern mathematicians who don’t understand that distinction any more.
Now, given any system typified by Euclidean geometry, in which you can prove propositions to be consistent (that is, consistent with one another), you can call these propositions “theorems.” Now, any system of theorems—which is sometimes called a “theorem-lattice”—can be shown to be underlain by a set of axioms and postulates. So, instead of thinking about the theorems, you can think about the set of axioms and postulates, because by implication, the set of axioms and postulates will describe, or identify, all of the theorems which are possible in that particular theorem-lattice.
Now, let’s state, in simple terms, exactly what it is that Riemann discovered. In March of 1853, a young genius by the name of Bernhard Riemann, who had studied at Göttingen and then Berlin and back to Göttingen, who had been a student and protégé of Carl Gauss, and also a student and protégé of Lejeune Dirichlet, made a discovery. And he was given special permission at the university to prepare for his habilitation dissertation as a professor by special research, whose purpose was to look through libraries and other sources, to see if there was any place in all science where something like his discovery had been elaborated.
A little over a year later, in June of 1854, he spent most of the day presenting his presentation and discussion in defense of it, to a group of professors at the university.
In brief, Riemann’s discovery can be described fairly and accurately as follows. His paper, “On the Hypotheses Which Lie at the Foundations of Geometry,” is one of the most beautifully written pieces in all scientific literature. There’s nothing obscure in it, it is clear; but almost nobody who has commented on it, has ever commented on it honestly, because it upsets all the mathematicians.
Let’s see what he attacked. He said that up to that point, there were problems in geometry, fundamental fallacies, which had been referred to by previous scientists, but whose implications had never really been defined. The only precedent he could find, of importance, was in two writings of Gauss. In Gauss’ first major publication at the end of the Eighteenth century, which is called Disquisitiones Arithmeticae, he deals with what are called “biquadratic residues,” which have to do with such things as prime number sequences, and things of that sort. And then, later on, Gauss wrote another paper, on the general theory of curved surfaces. Riemann identified these as the only two precedents that he could find for what he was trying to do.
Let me describe, in my own words, from the standpoint of theorem-lattices, what the problem was.
In what we call “Euclidean geometry,” people sometimes make the mistake of assuming that this Euclidean geometry—or Newtonian or Cartesian physics—has something to do with the real universe. In fact, they have nothing to do with the real universe. What we call “simple geometry” is not a creation of our senses: it is a creation of the imagination. We make some very simple assumptions. First, we make certain axiomatic assumptions, based on the imagination, about the nature of space and time. We assume that space is simply extended in three directions: forwards-backwards, up-down, and side-to-side. We assume that time is extended in one dimension, forwards-backwards. We assume that everything in space-time can be measured as “greater than” or “less than.”
Then we come along, and we try to put physics into space-time. We imagine that physical objects are based on objects like those we imagine we see, from our senses. We make two steps of assumptions about this. We imagine we put the object in space-time, and we do a kind of surveyor’s mapping of this object in space-time.
And then, we get more complicated. We let the object move in space-time, and we assume that the relations of measurement of objects in motion in simple space-time, have some correspondence to the relations of cause and effect in the real universe. We also introduce another assumption, which is the most dangerous and false assumption in all modern mathematical physics. It’s a fallacy, a falsehood which was defended vigorously by one of the most famous mathematicians of the Eighteenth century, a passionate—as a matter of fact, a fanatical—defender of Isaac Newton. He was a Swiss teacher of mathematics, who, through the patronage of Leibniz and Johann Bernoulli, was invited to Russia to the St. Petersburg Academy. In 1741, he was invited by one of the worst scoundrels in all Europe, Frederick II of Prussia, to move from St. Petersburg to the Academy at Berlin.
The Academy at Berlin was the center of hatred of Leibniz in Germany. It was the center for such degenerates as Pierre Louis Maupertuis, who was later kicked out of the Academy in 1753, because he had committed a great mathematical fraud. Also there at the time was Voltaire; and also a “pretty boy” from Italy called Francesco Algarotti, who was actually one of the sources for Immanuel Kant’s theory of aesthetics, was one of the controllers of science at the Berlin Academy at that time.
The gentleman whom I’m speaking of remained there from 1741, to about twenty-five years later, when he returned to the St. Petersburg Academy. He was responsible for a great number of useful contributions to mathematics, but also two of the greatest frauds in all mathematical history. His name was Leonhard Euler.
There were two issues here. First of all, Euler was part of the fraud that got Maupertuis kicked out of the Academy. Maupertuis claimed that he had discovered Leibniz’s theory of “least action.” So he was kicked out, because his fraud was so obvious. And Euler defended him, although Euler had worked enough with Leibniz’s work to know this was a fraud.
Euler’s great crime was published in 1761, in a paper called “Letters to a German Princess,” in which he attacked Leibniz’s Monadology, and in which he insisted that the continuity of space-time was infinitely, perfectly divisible [See Euler Box].
The importance of this consideration of Euler’s, which has many implications in the history of mathematics and physics, is that it becomes impossible to understand the relationship between mathematics and physics, and it becomes impossible to understand how scientific ideas affect the changes in productivity in society, for example.
What happens with a scientific discovery of principle? For me, the most popular example of this problem is one of the many important discoveries by a great man from the Third century B.C.E. This man, like many members of the Academy in Athens, Greece, came from Cyrenaica, which is an area now in Libya, on the southern coast of the Mediterranean. And his name was Eratosthenes, and the discovery I’m going to refer to, is his attempt to estimate the meridian of the Earth, which he measured to an accuracy of polar diameter of the Earth of about fifty miles error.
Let me describe the experiment to you. It’s a very simple one, but it illustrates some of the most fundamental problems in science [See Figure 1].
Eratosthenes’ method of measuring the size of the Earth
|
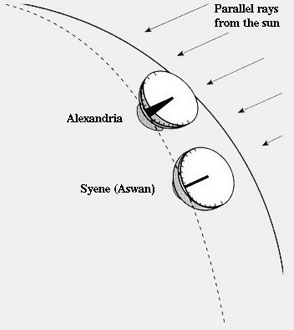 |
Here you are in Egypt near the end of the Third century B.C.E. You have no telescopes, you have only deep-well observations, and it will be 2,200 years before anybody will see the curvature of the Earth from space. How do you measure the size of the Earth, without leaving Egypt? What did he do?
Now, there’s a place which was called Syene, which is now under water, where the famous Aswan Dam is. There is the city of Alexandria, to the north. And if you were observing the stars, you could determine that Aswan is at a point approximately due south of Alexandria.
Now you make two sundials, with a special design. You take two hemispheres, you put a plumb bob (a weight on a string) on the bottom, and call it the South Pole of the hemisphere, to determine how to orient it. In the interior, from the South Pole up, you put a stick. And you grade the diameter of the sphere along the interior; you mark off equal segments along the line on the interior, which you intend to be your North-South line. Around the equator, you also make equal divisions. You make two of these sundials, and you put one in Syene (Aswan), and the other in Alexandria.
Obviously, the importance of using sundials, is that you want to make the observations at the same time of day in both places. So, for obvious reasons, you want to use noontime, when the sun is directly over the meridian. By using this method, you can determine that you are making your observations at the same time in Alexandria and at Syene, even though you have no radio, no telephone.
What do you observe? You observe the shadow of the sun cast by the stick, along the inside of your hemisphere. Now you compare the angles of the shadow in the two sundials. If the Earth were flat, the angles would be the same. If the Earth is not flat, the angles would not be the same. Obviously, they’re not the same. What do you do? You take the measurement of your angles, and you bring together your two measurements. You construct a circle, and so determine the angular distance between Syene and Alexandria. And, by comparing that with the length of the portion of the circumference of the circle it cuts off, you’ve estimated the size of the Earth.
Now, in teaching that experiment, which you obviously can know very easily, most modern schoolbooks or teachers would make a fundamental mistake. They would concentrate on the fact of the calculation, which is the least important part of the whole experiment. It’s very important, but it’s not the most important. The most important part of the experiment, given that it was not until 2,200 years later, that man for the first time saw the curvature of the Earth, is to ask a question: So how could someone in the Third century B.C.E. 2,200 years before anyone saw the curvature of the Earth, measure the curvature of the Earth to an accuracy of fifty miles diameter?
That’s the point. What did we measure? We did not measure what we saw. We measured an error in our observations, the difference between the two angles. So we created the idea of curvature we had never seen, by the contradiction shown in our experiment, a stubborn contradiction you could not remove.
Two things are demonstrated by that experiment. First of all, that knowledge is not based on experience. Knowledge is based on discovering the absurdities in our opinions about our experience. Science is based on those kinds of ideas which pertain to what we have not seen, but which we can then demonstrate to increase man’s power over nature.
Now let’s generalize that. We have three categories of the physical universe, in terms of our observation.
- We have the aspect of the universe which is within the range of our senses, or close to the range of our senses. That’s the ordinary macro-universe for us.
- Then we have a universe which we can see, but which we can’t see at the same time.
For example, Aristarchus, earlier in the same Third century B.C.E. was the first to demonstrate that the Earth orbited the sun. This was the work which, in the Second century A.C.E. a great fraudster studied. The fraudster’s name was Claudius Ptolemy. Claudius Ptolemy was an enthusiastic admirer of Aristotle, and he wished to discredit Aristarchus, and he wished to discredit the idea of Ideas, as Plato described Ideas. Remember, what I described as the Idea of the difference which enables us to understand curvature in Eratosthenes’ simple experiment, is the simplest example of what Plato meant by an Idea: a provable concept which does not depend upon direct observation.
Now, people like Ptolemy faked the data to say that the universe rotated around the Earth, and he made an absurd theory with faked data, to spread an idea, which was later overturned by Nicolaus of Cusa (you call him Nikolai Kuzansky), and then also later by Copernicus and Kepler. But this absurdity was widely believed in Europe.
Now, for Aristarchus, these observations involved estimated measurements of the distance from the Earth to the moon, which were reasonably accurate. They were wildly inaccurate, but for the observation, they were good ideas. And there were estimates of the distance from the Earth to the sun, which were much less accurate. This was done using eclipses.
And I cite these, because it is an example of a case in which mankind had never actually seen the distance between the Earth and the moon, or between the Earth and the sun; yet they were able to at least estimate a measurement. In fact, until we began to send out satellites and space rockets, we could never directly observe these relations. Yet, even in crude ways, in the time of the Greeks, these ideas of astrophysics existed. These are ideas of things we can’t see; but there are methods by which we can know them, which are, in principle, the same kind of method that was used by Eratosthenes to estimate the size of the Earth.
- Today, one of the most important areas of investigation, is an area to which we have no connection with our perceptual apparatus—the area of microphysics. We have no sense-perceptual direct access to any of this area, yet we have developed very precise and very efficient and useful ideas about this area. It’s in this area that the secrets of living processes, as well as the secrets of nuclear weapons, lie. With these methods, we can go down to distances of about 10 -18 centimeters. And the frontier is to go deeper.
So these are three categories of ideas which have nothing to do with “Euclidean geometry” in the ordinary sense.
Now, let’s take another experiment. As early as the beginning of the Sixteenth century, Leonardo da Vinci insisted that there was a finite rate of propagation of not only sound, but light; and, through the work of Kepler, this became very influential on a fellow called Christiaan Huyghens. Huyghens had a student called Øle Roemer, a Dane. These were all friends of Huyghens and of Leibniz, at the same Academy in Paris, under Colbert. Øle Roemer was a student there.
And Øle Roemer, in 1676, measured the speed of light, by making observations of the moons of Jupiter. His first estimate was very close to our modern one. On the basis of this, his teacher, Huyghens, developed a theory of refraction and reflection; because if light is propagated at a finite rate, this leads to certain conclusions.
Johann Bernoulli and Leibniz came up with a new estimate about the nature of the physical universe, which was based on the study of the behavior of the refraction of light, which is famously known as the brachistochrone problem, or least-time experiment. And so, on this basis, Leibniz and Johann Bernoulli attacked Descartes, and attacked Newton, and described the mechanical method, the mathematics of Newton and Descartes, to be incompetent, and said that, in mathematics, we must supersede algebra by a higher level of mathematics, which is called the mathematics of transcendental functions, which they also called, at times, non-algebraic functions.
So, this is a simple case of a discovery where physics, outside the domain of mathematics, began to force mankind to look at geometry in a new way. We had to change the axioms of assumption of geometry. This was something that had already been begun by the work of Kepler, who also thought of what we call today a quantized space-time rather than a continuous space-time.
And this is what Bernhard Riemann generalized, a whole series of experiments of this kind of impact. We find that every time we make a fundamental discovery of principle in physics, we create ideas of the type I described, Platonic ideas. These ideas force us to change the axioms of assumption which were used to create mathematics to describe physics. And this change of axioms gives to the appearance of space-time the character of a physical space-time curvature, and this is reflected as a difference in the way we measure relations within physical space-time.
Now, why was this important for me?
Every time you change an axiom in a theorem-lattice—call the old theorem-lattice A, and the new theorem-lattice B, where the difference between the two is a change in an axiom—no theorem of A will be consistent with B. You cannot, by any infinite approximation, ever reach B from A. This is called a discontinuity, or can be called, in certain cases, a singularity.
All human knowledge, including art, is based on this principle of discontinuity. It’s the fundamental difference between the mind of the human being and the animal. In art, we call this metaphor. You use language or painting or music to create a contradiction, a discontinuity. If you can show the discontinuity to be necessary, then it’s real. What is necessary, is real. Then this discontinuity, for which there is no word, becomes what we call a metaphor. A metaphor in art is the same thing as a discontinuity or singularity in scientific knowledge.
So, think about what you know. If you’ve studied well, you did not learn how to repeat the formula; you learned how to derive it. You did not simply copy any idea from someone because they were an authority; you learned how to repeat the act of discovery in your own mind.
Now, when you learn in that way, what you are doing is re-experiencing the mental act of discovery of people before you. You can be closer to Plato, than to your next-door neighbor, because you never visited the inside of your neighbor’s mind; but you’ve visited Plato’s mind. You can be closer to Beethoven, than to your marriage partner; because with your marriage partner, you never exchanged an idea.
Now, what do we know? Even in our use of language, what we have accumulated is discoveries by people thousands of years before us. All of these discoveries involve discoveries of principle, principles of what we call science and technology, principles of what we call art.
Now, what has happened to our minds as a result of the benefit we have received from our ancestors through a good education? Every discovery you have repeated in your mind has the representation of a discontinuity. The result is that we today can have in our minds more discontinuity for each individual act of thought, than our ancestors. Our thoughts are more powerful ideas, than those of our ancestors; and that is the source of the increase of the power of man over nature. And that’s why the famous theorem of Cantor about density of discontinuities per interval of action, is so important to me, and was so important to me back in 1952. It was the combined ideas of Cantor and Riemann which enabled me to understand the significance of the discovery I’d made in respect to information theory.
And that is an example of the relationship of philosophy and science to life. It is only an example, but perhaps you will find it more than enough to take in at one time.
Thank you.
* * *
|
Some Questions and Answers
|
Dr. Yuri Gromyko, Rector of the University: I would like to ask you a question from a rather different context. What do you think of the books of Alvin Toffler, who just now is rather popular?
This became known as “Project Air-Land Battle 2000,” which was used by a special unit of the U.S. forces to target an Iraqi tank division.
The idea was that American soldiers today would be stupid, because, I’ll tell you, the education system in the United States is not very good. It’s degenerated. But one thing young boys like to do, is to play electronic videogames for many hours after another.
So they got the idea: You put a helmet on this boy. He doesn’t see, he has this synthetic picture in his eyes; his ears are controlled by earphones; he wears electronic gloves, which give him sensations. And when he moves his fingers, they send signals which cause action. I don’t know about here, but in the United States, they have people go into these kinds of things: they put on these headsets, put these gloves on, and play videogames.
Now, imagine this little idiot in a tank. He’s wearing a headset, and he’s looking at the images coming by television, into these eyepieces. He sees an enemy tank, on the imager. His signal goes up to a satellite, which gives him a signal of what the tank’s position is, a radio-controlled rocket goes out, controlled by the satellite, to arrive at the precise position of the tank.
Now, what they came out and said is: “Ohhhhhhhhh!!! This is the new universe!”—of virtual reality.
Alvin Toffler was taken on as a propagandist for this project, and he began to write these silly books, thick books. Usually bad jokes should be short, shouldn’t they? But these are very long bad jokes! Actually, these jokes are based on gas theory. That’s why they’re so big.
Let me tell you, for example, who believes this nonsense. There’s a fellow called Lord William Rees-Mogg. He was former chief editor of the Times of London, and any high-ranking former Soviet spy will tell you that the London Times is the official organ for the London British oligarchy.
From the audience: Just like Pravda!
LaRouche: I don’t think Pravda ever perfected the art of lying the way the Times did.
So, he says the world is going to be a new kind of world. Ninety-five percent of the people will never receive any education at all. Wealth will be created by a few people, less than five percent, sitting on islands, dispensing information.
Now, let me just explain this. Because this is a significant question, I’ll give some background. If you include this crazy gas-theory of information, we know five different species of economic theory.
The first one, is the one which was perfected by Leibniz, which became the basis for the U.S. theory of economy.
The second one was based on Aristotle. It was called the Physiocratic doctrine. Macroeconomic profit was a new phenomenon in history—it did not exist as a social category until the Fifteenth century in Europe. So, everyone had to explain modern economy on the basis of this new phenomenon of the past five centuries, called macroeconomic profit, or surplus value.
Here’s how the Physiocrat François Quesnay, who was a Venetian agent, explained it. He said, “This comes from the bounty of nature. The Mother Earth goddess, Gaia, the patron goddess of prostitution, is the one who creates this wealth. It comes from forestry, it comes from agriculture, it comes from mining. It comes from the womb of Mother Gaia. Not from the peasants: the peasants are only human cattle, they’re like cows, you must feed them, but they don’t create anything.”
“But who does it belong to?”
“Oh, God gave the property title to the great lord. The state must not interfere, urban society must not interfere: laissez-faire.”
That was the theory of laissez-faire. Laissez-faire theory says that good comes only from evil, that the interaction of the evil acts of individual persons results in a “gas-theory”-like equilibrium, an equilibrium among evil acts, and that the equilibrium is good. That’s the theory of laissez-faire.
Then you have a third one, which came after that. Adam Smith went to study with the students of the Physiocrats Quesnay and Turgot in France. He was an agent of the British East India Company. He came back and he copied the theory, calling it laissez-faire “free trade.” But he said “No, it is not nature that creates wealth; it is trade that gives wealth.”
Fourth: Marx studied this (he made one slight improvement, which is called the theory of social reproduction, but otherwise he copied these fellows), and made one slight change. He said surplus value comes from labor, which became known as the labor theory of value. Then Engels added a stupid mistake. Seeing the hands of the British apes—the British royal family—Engels saw the opposable thumb. So he said the mechanical action of the opposable thumb creates technology as an epiphenomenon of the movement of the thumb.
Then, fifth, along come Von Neumann and company, and these fellows say, “No. Wealth comes from information,” and it is simply a result of what they call negentropy, which is a reversal of entropy, in the human gas system. So, what happens? Today Lord Rees-Mogg comes up with this theory, which is a new version of Aristotelian metaphysics. It’s a form of superstition to say that an object by its nature “secretes” something.
But if you look as I do at what I described, you’d look at society and you’d say, “Let’s describe the society in terms of very simple thermodynamics. Let’s take two kinds of things. First, in terms of consumption by people, by households, by industry. Let’s call these market-baskets.”
Now, this market basket contains the physical things we consume, or industry or farmers must consume. It includes things like the production of power, and the production of water and transportation. It includes services and education. It includes health care. It includes science as such. These are the things which are essential to the productivity of people and of society.
Second, let’s compare what people consume, and what society consumes, with what society produces. Let’s compare the things we consume, with the same kind of things we produce.
In order to maintain society at a certain level of productivity and technology, we find that we can write bills of materials and process sheets which describe the requirements to do that. We can do that. That requirement, which we’ve determined, is the energy of the system. We measure the energy of the system per capita of the labor force, by the household, and by the square kilometer of land used. So we get a notion of energy density.
Now we compare consumption with production, of the same things. We make an allowance for the administration of society. We come out with what we may call the excess, or the free energy.
There are two things to consider. The first thing you’re interested in, is the ratio of the free energy to the energy of the system, comparing these as a whole, and comparing it per capita of the labor force, per household (because we breed children in households), and per unit of land area.
Now, we’re concerned with the ratio of free energy to energy. Well, what should we do with the free energy? We should invest it in society’s improvement, which means the energy of the system per capita will increase. So now we have more energy of the system per capita, per square kilometer. But we want the ratio of the free energy to energy of the system not to fall, when the energy of the system per capita increases. In society, that’s what we call capital intensity, energy intensity.
In other words, the requirement of success in an economy, is that the rate of growth should not fall with the increase of the capital intensity.
Therefore, what do you have? You have, on the one hand, this kind of process I’ve described, and it is not-entropic. This is not the negentropy of Boltzmann and Wiener, or Toffler. This is a not-entropy.
What causes the not-entropy of society? The human species is the only species in which this behavior exists. Not-entropy exists in the biosphere, but only in the biosphere, not in the individual species. Through evolution, the biosphere achieves higher states. But only human beings, only society, can increase its not-entropy by its own will—what I described before, the not-entropy of increased density of discontinuities. You can say that the rate of scientific discovery, and the rate at which society uses them, typifies—that is, it’s not the exclusive cause of, but it’s the typical cause of—the increase in the not-entropy of the economy.
The greatest achievement of economy in the former Soviet Union was in the military-industrial-scientific sector. The driver of that success was science as such, and the derivatives of scientific work in engineering, which is not-entropic. The problem was that the lack of infrastructure development and the lack of emphasis on this in the civilian economy under conditions of arms race, prevented that benefit from spilling into the civilian economy.
So, when you look at Toffler’s work, you say: “This is idiocy.”
What we have to do, is to educate our children better, to eliminate textbook education, and have the students instead relive the derivation of these discoveries. Educate every child as if that child were going to be a genius, and you will have a good society—and you will also have many geniuses. Then it will work.
Nina Gromyko: Could I interpolate a question here? Do you have, so to speak, an elaborated educational technology? Do you have some form in which you can bring children into this world of discovery?
Lyndon LaRouche: There are two things involved. First of all, I would start with the Classical Greeks, in terms of science. And there are certain things that are obvious: You always teach the concept which is necessary before the next concept, which depends upon the first. One discovery is the precondition for the next discovery, and the main thing is this experimental process, where the student actually relives the act of discovery. So the class size should not be too great, because the student must not only do his own individual work, but there must be discussion, a Socratic type of discussion, in order that the digestion of this activity is made conscious by discussing it. The child should learn great experiments, as rapidly as the child can go from one to the next level.
Once the student gets the habit of learning that way, in the classroom, that way of thinking becomes a habit of life. Most of what people learn, is learned outside school. But the educational system provides the skeleton and the ability for the person to do this activity outside the classroom. And the asking of the right questions and the discussion of the ideas in the classroom, is the process by which this is digested.
Nina Gromyko: We thank you very much for your presentation here. A lot of what you put forward is very close to us, but the question also arises of how to generate practical forms for bringing these ideas to life before various audiences, both children and adults. Thank you very much, once again.
|
back to article
Euler Box
|
|
Footnotes |
||
![]()
schiller@schillerinstitute.org
The Schiller Institute
PO BOX 20244
Washington, DC 20041-0244
703-297-8368
Thank you for supporting the Schiller Institute. Your membership and contributions enable us to publish FIDELIO Magazine, and to sponsor concerts, conferences, and other activities which represent critical interventions into the policy making and cultural life of the nation and the world.
Contributions and memberships are not tax-deductible.
VISIT THESE OTHER PAGES:
Home | Search | About | Fidelio | Economy | Strategy | The LaRouche Frameup | Conferences
Links | LaRouche | Music | Join | Books | Concerts | Highlights | Education |
Health | Spanish Pages | Poetry | Dialogue of Cultures
Maps | What's New
© Copyright Schiller Institute, Inc. 2006. All Rights Reserved.